Water is flowing out of a hole in the bottom of a cylindrical container. The initial height of the water in the container is $0.6\text{m}.$

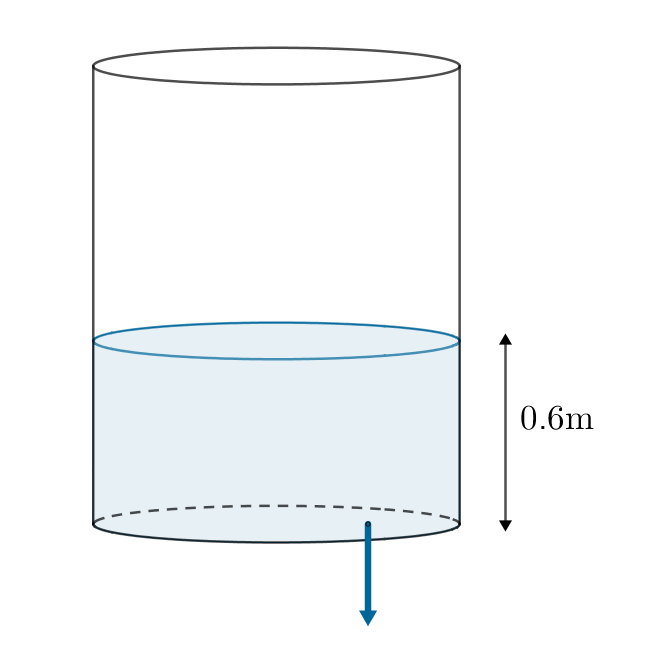
As the cylinder empties, the water flows out less quickly, so that

1. Given that the cylinder empties after $4$ seconds, use a Riemann sum with intervals of width $0.5\text{s}$ to work out an estimate for the radius of the cylinder.

2. Using $\int$ notation, write down an expression which represents the exact radius of the cylinder.

Give your estimate for the radius, correct to $3$ significant figures.